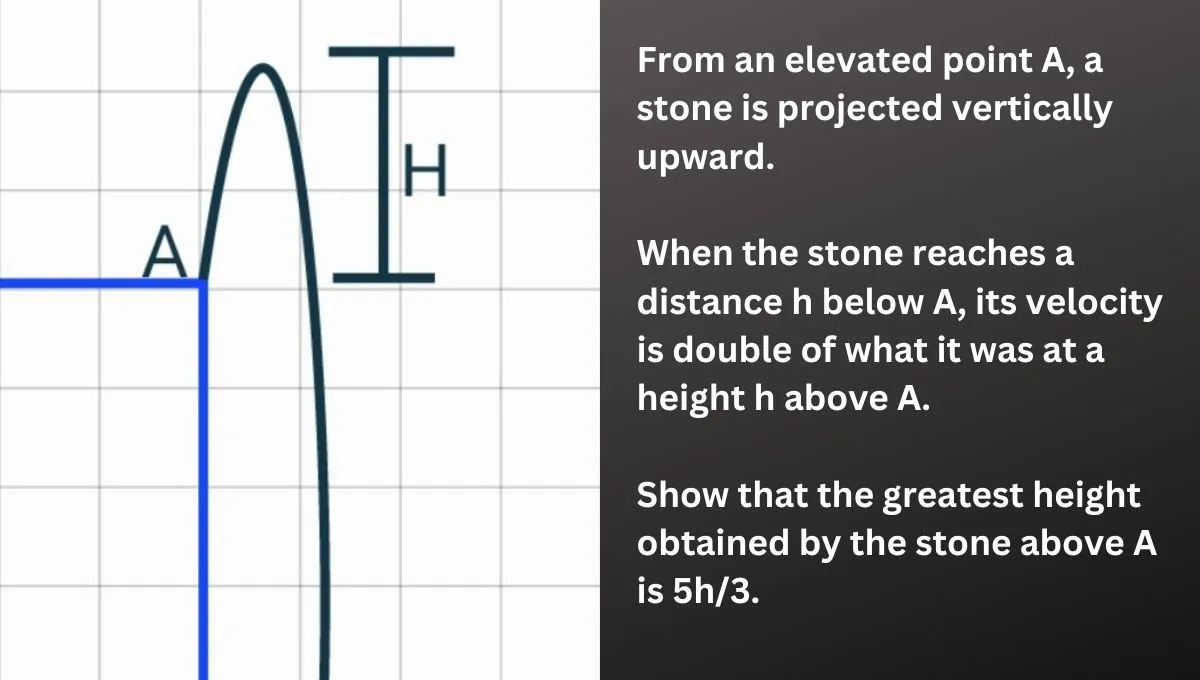
From an elevated point A, a stone is projected vertically upward. When the stone reaches a distance h below A, its velocity is double of what it was at a height h above A. Show that the greatest height obtained by the stone above A is ![]() .
.
| Property | Description (Based on the Article Title) |
| Object | Stone |
| Initial Position | Elevated point A (specific height might be given in the article) |
| Motion | Vertically upward (one-dimensional) |
| Focus | * Behavior at a specific height relative to the starting point (h) * Relationship to the maximum height reached |
| Provided Information | * The stone’s velocity at a height h below the starting point (might be double the velocity at h above it) |
| Solved Property | The maximum height reached by the stone above point A |
The problem describes the motion of a stone thrown vertically upward under the influence of gravity from an elevated point A. At a certain instance during its ascent and subsequent descent, the stone’s velocity at a distance h below point A is twice what it was at a height h above point A. The goal is to determine the maximum height the stone reaches above the starting point A.
Explanation:
The motion of the stone under gravity is a one-dimensional motion with a constant acceleration due to gravity (g). Using kinematic equations and the conservation of mechanical energy, the velocities at different heights can be related to each other. The stone’s kinetic energy decreases as it ascends due to work done against gravity and increases as it descends, with the potential energy converting back into kinetic energy.
Solution Steps:
Kinematic Equations for Vertical Motion:
The stone’s initial velocity at the launch point (point A) is unknown and denoted by u.
At height h above point A, the stone’s velocity decreases due to gravity:
![]() (Equation 1).
(Equation 1).
At height h below point A, the stone’s velocity increases as it falls back down:
![]() (Equation 2).
(Equation 2).
According to the problem statement, the velocity at h below A is twice that at h above A:
![]() (Equation 3).
(Equation 3).
Squaring both the sides
![]()
Substitute ![]() from Equation 1 and
from Equation 1 and ![]() from Equation 2 into Equation 3 :
from Equation 2 into Equation 3 :
![]()
![]()
![]() (Equation 4)
(Equation 4)
Calculating Maximum Height (H):
The maximum height is achieved when the stone’s velocity is zero.
The kinematic equation for the ascent is:
![]()
![]()
Inserting ( u^2 ) from Equation 4 into this last equation to solve for H in terms of h.
![]()
![]()
Final Answer:
The maximum height H reached by the stone above point A is ![]() . This result is obtained through the application of kinematics for vertical motion under the influence of gravity.
. This result is obtained through the application of kinematics for vertical motion under the influence of gravity.