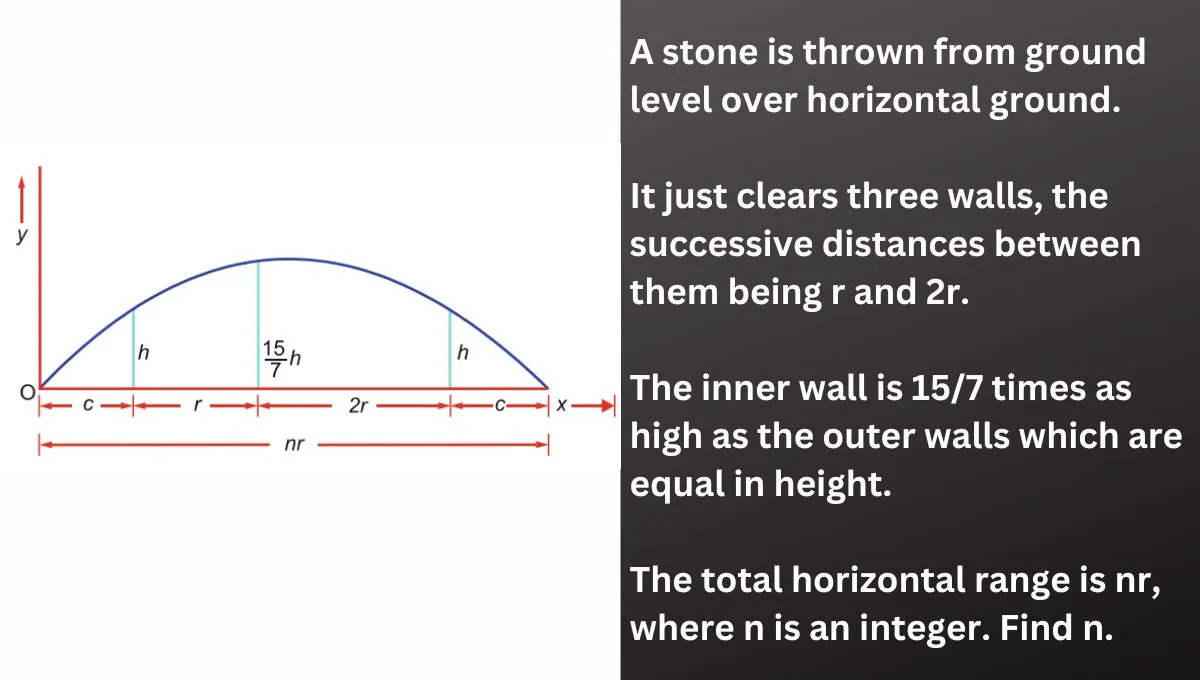
A stone is thrown from ground level over horizontal ground. It just clears three walls..

Spread the love

A stone is thrown from ground level over horizontal ground. It just clears three walls, the successive distances between them being r and 2r. The inner wall is 15/7 times as high as the outer walls which are equal in height. The total horizontal range is nr, where n is an integer. Find n.

Projectile	Stone
Motion	Horizontal
Walls Cleared	3
Wall Height	Middle wall is the tallest
Solved Property	Total horizontal distance traveled
Equation	Derived in the article
Conclusion	Total horizontal distance is four times the distance between the successive walls

The problem presented is a practical application of projectile motion in physics. A stone is thrown horizontally from the ground level and it clears three walls of varying heights, with the first and last walls being of equal height, and the middle wall being taller. The stone follows a parabolic trajectory, which is characteristic of projectiles under the influence of gravity.

Explanation: Projectile motion can be broken down into horizontal and vertical components. The horizontal motion is uniform since there is no acceleration in that direction, and the vertical motion is subject to gravitational acceleration. The path of the projectile is parabolic, and the equation of its trajectory can be represented as $y = x \tan(\theta) \left(1 - \frac{x}{R}\right)$ , where $y$ is the vertical height, $x$ is the horizontal distance covered, $\theta$ is the angle of projection, and $R$ is the range of the projectile.

Given that $R = nr$ and the stone clears walls at distances $c$ , $c+r$ , and $c+3r$ , with the heights of the outer walls being $h$ and the inner wall $\frac{15}{7}h$ , we can use the provided equation to set up a system of equations based on the stone’s height at these three positions:

At the first wall (distance $c$ ), the height is $h$ :
$h = c \tan(\theta) \left(1 - \frac{c}{nr}\right)$
At the second wall (distance $c+r$ ), the height is $\frac{15}{7}h$ :
$\frac{15}{7}h = (c+r) \tan(\theta) \left(1 - \frac{c+r}{nr}\right)$
At the third wall (distance $c+3r$ ), the height is again $h$ :
$h = (c+3r) \tan(\theta) \left(1 - \frac{c+3r}{nr}\right)$

Since the range $R$ is also given by $R = c + r + 2r + c$ , it simplifies to $R = 2c + 3r$ . From this, we can express $c$ in terms of $n$ and $r$ :

$2c + 3r = nr$

$2c = (n-3)r$

$c = \frac{(n-3)r}{2}$

We can now substitute this expression for $c$ into our equations for the height at the three walls and solve for $n$ by equating the heights at the outer walls and incorporating the height at the second wall. This will give us a system of equations involving $h$ , $r$ , $n$ , and $\theta$ , which we can solve to find the value of $n$ .

To solve for $n$ , as suggested, we’ll divide equation 2 by equation 1, and then substitute $c$ with $\frac{(n-3)r}{2}$ .

From the equations:

$h = c \tan(\theta) \left(1 - \frac{c}{nr}\right) \quad \text{(equation 1)}$

$\frac{15}{7}h = (c+r) \tan(\theta) \left(1 - \frac{c+r}{nr}\right) \quad \text{(equation 2)}$

Dividing equation 2 by equation 1:

$\frac{\frac{15}{7}h}{h} = \frac{(c+r) \tan(\theta) \left(1 - \frac{c+r}{nr}\right)}{c \tan(\theta) \left(1 - \frac{c}{nr}\right)}$

Simplifying this gives:

$\frac{15}{7} = \frac{(c+r) \left(nr - c - r\right)}{c(nr - c)}$

Now we substitute $c$ with $\frac{(n-3)r}{2}$ :

$\frac{15}{7} = \frac{\left(\frac{(n-3)r}{2} + r\right) \left(nr - \frac{(n-3)r}{2} - r\right)}{\frac{(n-3)r}{2}\left(nr - \frac{(n-3)r}{2}\right)}$

$\frac{15}{7} = \dfrac{n^2 - 1}{n^2 - 9}$

$15n^2 -135 = 7n^2 - 7$

$8n^2 =128$

$n^2 = 16$

We find two possible solutions for $n$ : -4 and 4.

However, since $n$ represents a positive range in this context (the total horizontal distance the projectile travels), the negative value does not make physical sense. Therefore, the appropriate value for $n$ that satisfies the conditions of the problem is $n = 4$ . This means that the total horizontal range $R$ is 4 times the distance $r$ between the successive walls.