A thin cylindrical wheel of radius r = 40 cm is allowed to spin on a frictionless axle. The wheel, which is initially at rest, has a tangential force applied at right angles to its radius of magnitude 50 N. The wheel has a moment of inertia equal to 20 kg m^2...[latexpage]

Spread the love

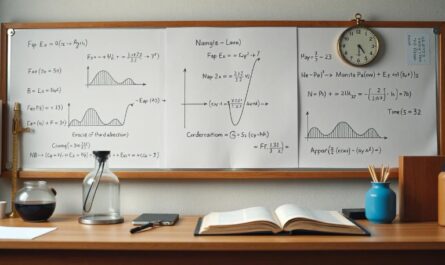
(a) A thin cylindrical wheel of radius r = 40 cm is allowed to spin on a frictionless axle. The wheel, which is initially at rest, has a tangential force applied at right angles to its radius of magnitude 50 N. The wheel has a moment of inertia equal to $20 kg m^2$ .
Calculate
(i) The torque applied to the wheel
(ii) The angular acceleration of the wheel
(iii) The angular velocity of the wheel after 3 s
(iv) The total angle swept out in this time
(b) The same wheel now has the same force applied but inclined at an angle
of $20^\circ$ to the tangent. Calculate
(i) The torque applied to the wheel
(ii) The angular acceleration of the wheel

Here is an informative table summarizing the solution for both parts (a) and (b):

Part	Physical Quantity	Formula	Calculation	Answer
(a)	Torque ( $\tau)$	$\tau = r \times F$	$0.4 \, \text{m} \times 50 \, \text{N}$	$20 \, \text{Nm}$
	Angular Acceleration ( $\alpha$ )	$\alpha = \frac{\tau}{I}$	$\frac{20}{20}$	$1 \, \text{rad/s}^2$
	Angular Velocity ( $\omega$ ) after 3 s	$\omega = \omega_0 + \alpha t$	$0 + (1 \, \text{rad/s}^2) \times 3$	$3 \, \text{rad/s}$
	Total Angle ( $\theta$ )	$\theta = \omega_0 t + \frac{1}{2} \alpha t^2$	$0 + \frac{1}{2} \times 1 \times 3^2$	$4.5 \, \text{rad}$
(b)	Torque ( $\tau$ )	$\tau = r \times F \times \cos(\theta)$	$0.4 \times 50 \times \cos(20^\circ)$	$18.794 \, \text{Nm}$
	Angular Acceleration ( $\alpha$ )	$\alpha = \frac{\tau}{I}$	$\frac{18.794}{20}$	$0.9397 \, \text{rad/s}^2$

In this problem, a tangential force is applied to a wheel, and we need to calculate torque, angular acceleration, angular velocity, and total angle. This requires using fundamentals of rotational dynamics such as torque $(\tau)$ , angular acceleration $(\alpha)$ , and angular velocity $(\omega)$ .

Explanation: Torque is a measure of the ability to rotate an object about an axis. It is expressed by the following formula:

$\tau = r \times F \times \sin(\theta)$

where:

$r$ is the radius of the wheel.
$F$ is the value of the force.
$\theta$ is the angle between the force and the radius.

Angular acceleration $(\alpha)$ is the rate of change of angular velocity, which is obtained from the following formula:

$\tau = I \alpha$

where (I) is the moment of inertia. Angular velocity $(\omega)$ can be found from the following formula:

$\omega = \omega_0 + \alpha t$

Angular displacement ( $\theta$ ) is used for:

$\theta = \omega_0 t + \frac{1}{2} \alpha t^2$

Solution:

(a) Part

Context: A thin cylindrical wheel has a radius $r = 40 \, \text{cm} = 0.4 \, \text{m}$ on which a tangential force of $F = 50 \, \text{N}$ is applied perpendicular to its radius. The moment of inertia of the wheel is $I = 20 \, \text{kg m}^2$ . The wheel is initially at rest.

Explanation and Solution:

(i) Torque applied to the wheel
Torque ( $\tau$ ) is calculated as:

$\tau = r \times F$

$\tau = 0.4 \, \text{meter} \times 50 \, \text{N} = 20 \, \text{Nm}$

Answer:
$\tau = 20 \, \text{Nm}$

(ii) Angular acceleration of the wheel
Using $\tau = I \alpha$ :

$20 \, \text{Nm} = 20 \, \text{kg m}^2 \times \alpha$

$\alpha = \frac{20}{20} = 1 \, \text{rad/second}^2$

Answer:
$\alpha = 1 \, \text{rad/second}^2$

(iii) Angular velocity after 3 seconds
Using $\omega = \omega_0 + \alpha t$ :

$\omega = 0 + (1 \, \text{rad/second}^2) \times 3 \, \text{seconds}$

$\omega = 3 \, \text{rad/second}$

Answer:
$\omega = 3 \, \text{rad/second}$

(iv) Total angular displacement
Using $\theta = \omega_0 t + \frac{1}{2} \alpha t^2$ :

$\theta = 0 + \frac{1}{2} \times 1 \times (3 \, \text{sec})^2$

$\theta = \frac{1}{2} \times 9 = 4.5 \, \text{rad}$

Answer:
$\theta = 4.5 \, \text{rad}$

(b) Part

Now the same force $F = 50 \, \text{N}$ is applied, but at an angle of $20^\circ$ to the tangent. The moment of inertia of the wheel is $I = 20 \, \text{kg m}^2$ .

Explanation and Solution:

(i) Torque applied on the wheel

Since the force is applied at an angle, the torque is given by:

$\tau = r \times F \times \cos(\theta)$

$\tau = 0.4 \, \text{m} \times 50 \, \text{N} \times \cos(20^\circ)$

First find the value of $\cos(20^\circ) \approx 0.9397$ :

$\tau = 0.4 \times 50 \times 0.9397 = 18.794 \, \text{Nm}$

Answer:
$\tau = 18.794 \, \text{Nm}$

(ii) Angular acceleration of the wheel
$\tau = I Using \alpha$ :

$18.794 \, \text{Nm} = 20 \, \text{kg m}^2 \times \alpha$

$\alpha = \frac{18.794}{20} = 0.9397 \, \text{rad/second}^2$

Answer:
$\alpha = 0.9397 \, \text{rad/second}^2$

Final Answer

(a)
(i) $\tau = 20 \, \text{Nm}$
(ii) $\alpha = 1 \, \text{rad/second}^2$
(iii) $\omega = 3 \, \text{rad/second}$
(iv) $\theta = 4.5 \, \text{rad}$

(b)
(i) $\tau = 18.794 \, \text{Nm}$
(ii) $\alpha = 0.9397 \, \text{rad/second}^2$