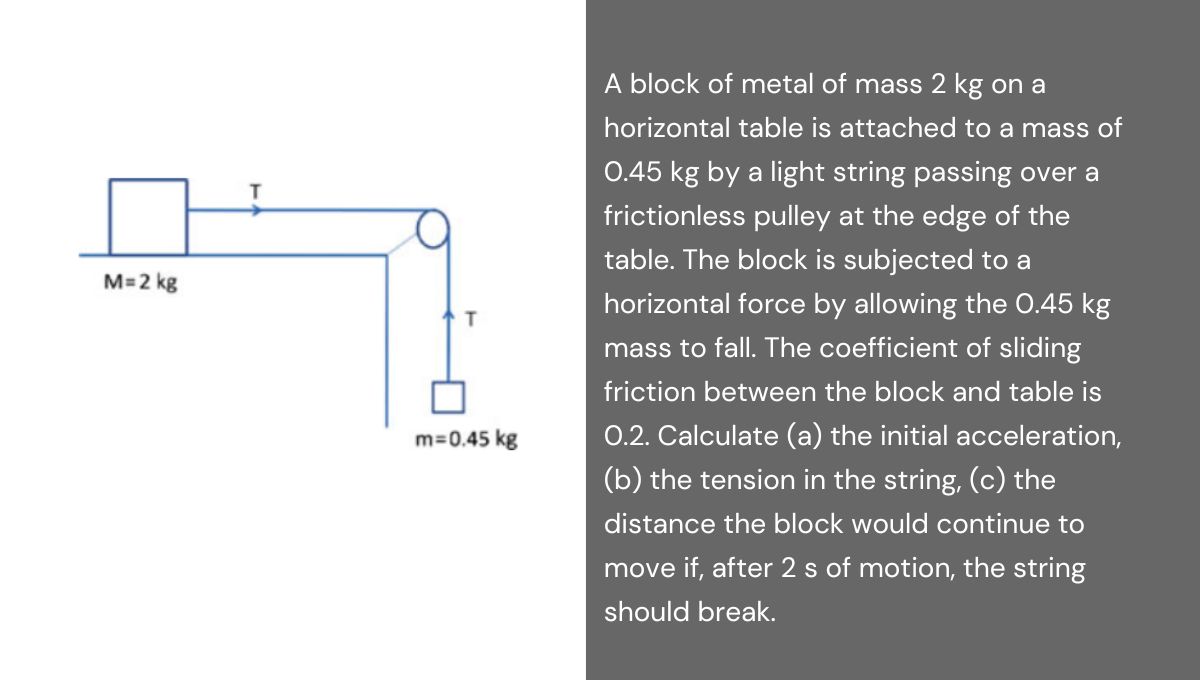
A block of metal of mass 2 kg on a horizontal table is attached to a mass of 0.45 kg by a light string passing over a frictionless pulley at the edge of the table. The block is subjected to a horizontal force by allowing the 0.45 kg mass to fall. The coefficient of sliding friction between the block and table is 0.2. Calculate (a) the initial acceleration, (b) the tension in the string, (c) the distance the block would continue to move if, after 2 s of motion, the string should break.
In this question, a metal block of mass 2 kg is placed on a horizontal table and is connected to a smaller mass of 0.45 kg by a light rope. The rope passes over a frictionless pulley. When the smaller mass is allowed to fall, it pulls the 2 kg block horizontally across the table. The coefficient of friction between the block and the table is given as 0.2. The goal is to:
(a) Find the initial acceleration of the block and the falling mass.
(b) Calculate the tension in the rope.
(c) Find the distance the block will travel after 2 seconds of motion, when the rope should break.
Explanation:
- First, consider the forces acting on the block
 and the smaller mass
and the smaller mass  .
. - For the block, the horizontal force is the tension in the rope
 , while the friction force
, while the friction force  opposes the motion. Here
opposes the motion. Here  is the coefficient of friction, and
is the coefficient of friction, and  is the normal force, which is equal to the weight of the block
is the normal force, which is equal to the weight of the block  .
. - For the smaller mass, the pulling force is the gravitational force ( m \times g ), and the tension of the rope
 acts in the upward direction.
acts in the upward direction. - Using Newton’s second law, the motion equations for both the masses can be written and solved simultaneously to find the value of acceleration
 and tension
and tension  .
. - Finally, using the kinematic equations, the distance covered in 2 seconds can be calculated.
Solution:
- For the block
 on the table:
on the table:
The friction force is
![]()
The net force on the block is
- For the hanging mass
 :
:
The net force on the hanging mass is .
.
Using the equations of motion of both the masses:
![]()
![]()
Substituting the values in these equations:
From equation (1):
![]()
From equation (2):
![]()
Substituting ![]() in:
in:
![]()
Solving for
![]()
![]()
![]()
- Tension in the rope:
Using ![]() :
:
![]()
- Distance travelled in 2 seconds:
Using the equation ![]() (initial velocity ( u = 0 Using )):
(initial velocity ( u = 0 Using )):
![]()
![]()
Final Answer:
- (a) Initial acceleration
 .
. - (b) Tension in the rope
 .
. - (c) Distance traveled
 .
.


![An infinitely long wire, located on the z-axis, carries a current \( I \) along the \( +z \)-direction and produces the magnetic field \( \vec{B} \). The magnitude of the line integral \( \int \vec{B} \cdot d\vec{l} \) along a straight line from the point \( (-\sqrt{3}a, a, 0) \) to \( (a, a, 0) \) is given by \[ \text{[} \mu_0 \text{ is the magnetic permeability of free space.] } \]](https://physicsqanda.com/wp-content/uploads/2025/04/resized-image-1-445x265.jpg)