(a) A tiny ball of mass 0.6 g carries a charge of magnitude 8μC. It is suspended by a thread in a downward electric field of intensity 300N/C. What is the tension.....

Spread the love

Contents hide

Problem:

(a) A tiny ball of mass $0.6$ g carries a charge of magnitude $8\mu$ C. It is suspended by a thread in a downward electric field of intensity $300$ N/C.
What is the tension in the thread if the charge on the ball is
(i) positive?
(ii) negative?
(b) A uniform electric field is in the negative x-direction. Points a and b are on the x-axis, a at x = 2m and b at x = 6m.
(i) Is the potential difference $V_b - V_a$ positive or negative?
(ii) If the magnitude of $V_b - V_a$ is $10^5$ V, what is the magnitude E of the electric field?

Explanation:

Part (a):
The forces acting on the ball are:

Gravitational force, 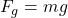$F_g = mg$ , acting downward.
Electric force, 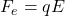$F_e = qE$ , acting downward for a positive charge and upward for a negative charge.
The tension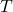 $T$ in the thread is determined by balancing the forces.

For a positive charge, the tension is:

$T = F_g + F_e$

For a negative charge, the tension is:

$T = F_g - F_e$

Part (b):
The relationship between electric field $E$ , potential difference $\Delta V = V_b - V_a$ , and distance $d$ is:

$E = \dfrac{|\Delta V|}{d}$

The potential difference ( V_b – V_a ) is positive because the electric field points in the negative x-direction, and the potential decreases in the direction of the field.

Solution:

Part (a):

(i) For a positive charge:
The gravitational force is:

$F_g = mg = (0.0006)(9.8) = 0.00588 \, \text{N}$

The electric force is:

$F_e = qE = (8 \times 10^{-6})(300) = 0.0024 \, \text{N}$

The tension is:

$T = F_g + F_e = 0.00588 + 0.0024 = 0.00828 \, \text{N}$

(ii) For a negative charge:
The tension is:

$T = F_g - F_e = 0.00588 - 0.0024 = 0.00348 \, \text{N}$

Final Answers (a):
(i) $T = 0.00828 \, \text{N}$
(ii) $T = 0.00348 \, \text{N}$

Part (b):

(i) Sign of $V_b - V_a$ :
The electric field points in the negative x-direction, and the potential decreases along the field’s direction. Therefore, ( V_b – V_a ) is positive.

(ii) Magnitude of the electric field:
The distance between $a$ and $b$ is: