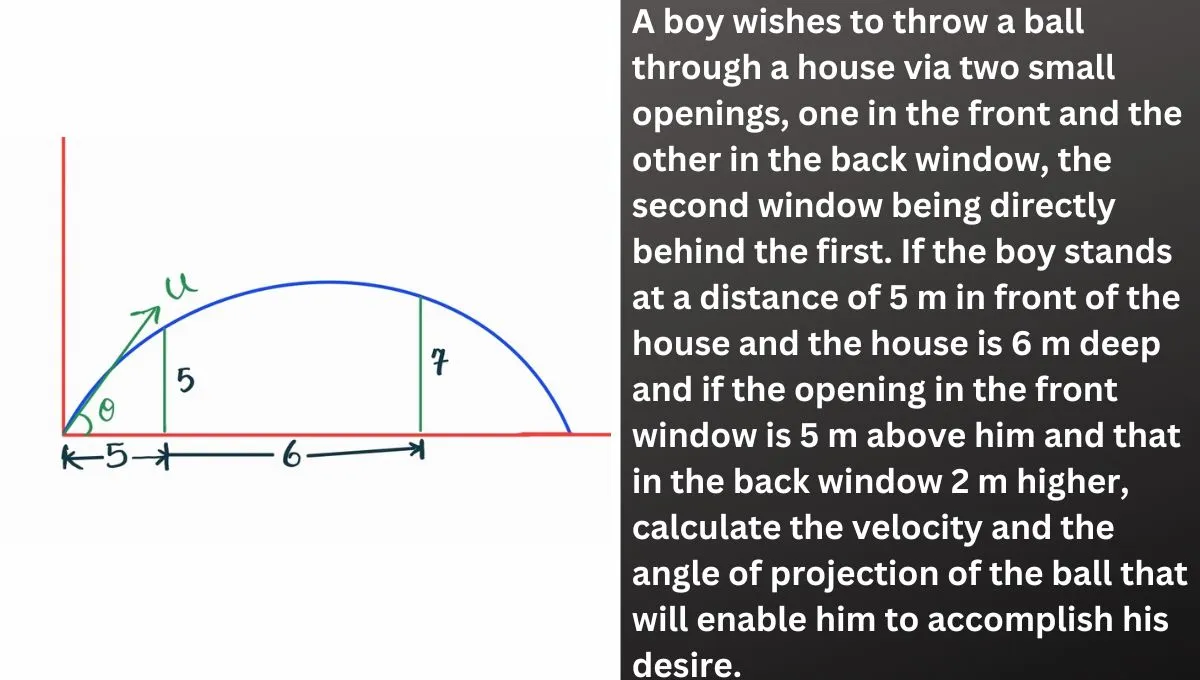
A boy wishes to throw a ball through a house via two small openings, one in..

Spread the love

A boy wishes to throw a ball through a house via two small openings, one in
the front and the other in the back window, the second window being directly
behind the first. If the boy stands at a distance of 5 m in front of the house and
the house is 6 m deep and if the opening in the front window is 5 m above him
and that in the back window 2 m higher, calculate the velocity and the angle
of projection of the ball that will enable him to accomplish his desire.

Factor	Description
Window Size	The size of the windows needs to be large enough for the ball to pass through without clipping the edges.
Ball Trajectory	The ball needs to be thrown with a trajectory that ensures it passes through both windows.
Throwing Distance	The distance from where the throw is initiated will affect the launch angle and velocity needed.
Window Height	The relative heights of the windows play a role in determining the trajectory. Identical heights simplify calculations.
Ballistics	Understanding projectile motion principles is crucial for calculating the launch angle and velocity.
Air Resistance	While negligible for short throws indoors, air resistance can slightly affect the trajectory in real-world scenarios.

The scenario described is an application of projectile motion, a type of motion experienced by an object that is thrown near the Earth’s surface and moves along a curved path under the action of gravity only. The problem involves determining the initial velocity and angle of projection for a ball to pass through two windows of a house, with specific constraints on the distances and heights of the windows.

Contents hide

1 Explanation:

2 Solution:

3 Final Answer:

Explanation:

Projectile motion can be characterized by a parabolic path, described by the equation $y = ax - bx^2$ , where $y$ is the height, $x$ is the horizontal distance, $a$ and $b$ are constants related to the angle and velocity of projection. The angle $\theta$ and initial velocity $u$ can be found by solving for $a$ and $b$ using the given coordinates of the openings in the windows.

Solution:

Taking the point of projection as the origin, the coordinates of the two points $(5, 5)$ and $(11, 7)$ , which represent the $(x, y)$ coordinates of the openings in the windows, two equations can be established from the equation of the parabolic path:

$5 = 5a - 25b$ (3)
$7 = 11a - 121b$ (4)

These are two simultaneous equations with two unknowns $a$ and $b$ . Solving these equations will give the values of $a$ and $b$ , which can then be used to find $\theta$ and $u$ .

Also solve this on projectile

First, solve equation (3) for $a$ :
$a = \dfrac{5 + 25b}{5}$

Next, substitute $a$ from equation (3) into equation (4):
$7 = 11\left(\dfrac{5 + 25b}{5}\right) - 121b$

Simplify and solve for $b$ :
$7 = 11 + 55b - 121b$
$7 = 11 - 66b$
$-4 = -66b$
$b = \dfrac{4}{66}$
$b = 0.0606$

Now, use the value of $b$ to solve for $a$ :
$a = \dfrac{5 + 25(0.0606)}{5}$
$a = \dfrac{5 + 1.515}{5}$
$a = \dfrac{6.515}{5}$
$a = 1.303$

With $a$ and $b$ found, use the given equations $a = \tan(\theta)$ and $b = \dfrac{g}{2u^2\cos^2(\theta)}$ to solve for $\theta$ and $u$ , where $g$ is the acceleration due to gravity (approximately $9.8 \, m/s^2 )$ .

First, find $\theta$ using $a = \tan(\theta)$ :
$\tan(\theta) = 1.303$
$\theta = \arctan(1.303)$
$\theta \approx 52.5^\circ$

Next, solve for $u$ using $b = \dfrac{g}{2u^2\cos^2(\theta)}$ :
$0.0606 = \dfrac{9.8}{2u^2\cos^2(52.5)}$

To find $u$ , rearrange the equation:
$u^2 = \dfrac{9.8}{2(0.0606)\cos^2(52.5)}$

First, calculate the denominator:
$\cos(52.5) \approx 0.6$
$2(0.0606)(0.6)^2 \approx 0.043632$

Now, solve for $u^2$ :
$u^2 = \dfrac{9.8}{0.043632}$
$u^2 \approx 224.48$
$u \approx \sqrt{224.48}$
$u \approx 14.98 \, m/s$

Final Answer:

The velocity and angle of projection required for the ball to pass through both windows are approximately $u = 14.98 \, m/s$ and $\theta = 52.5^\circ$ .

Explanation:

Solution:

Final Answer:

Related Posts

Leave a Comment Cancel Reply