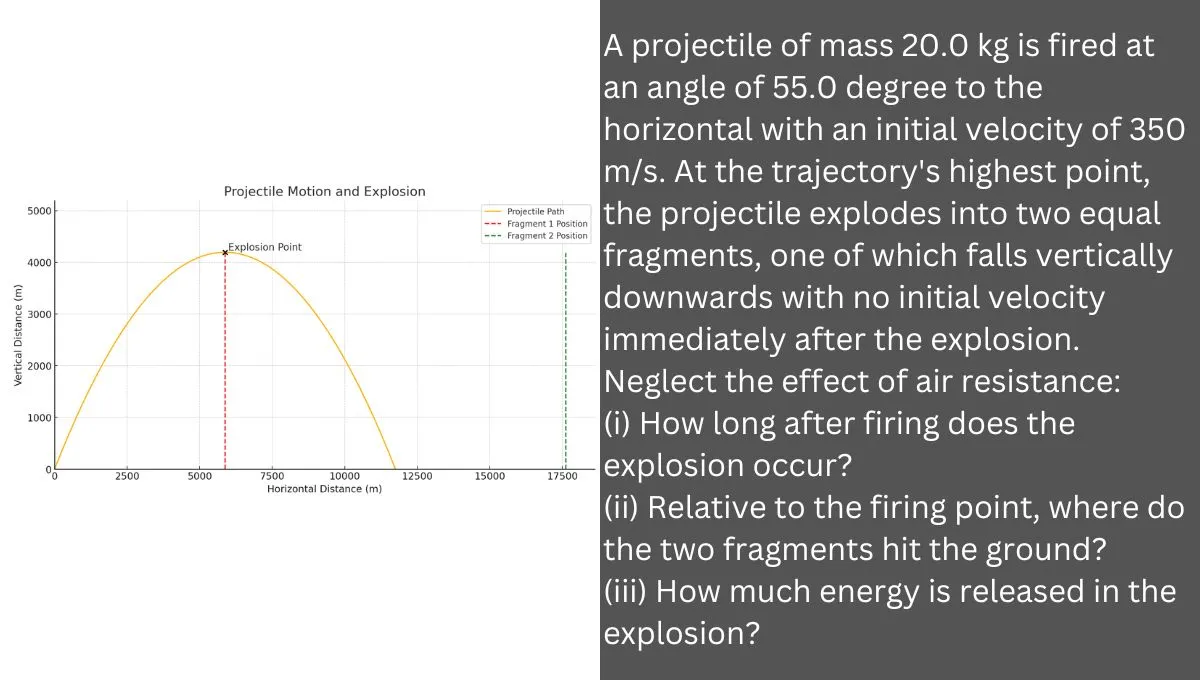
A projectile of mass 20.0 kg is fired at an angle of (Large 55.0^circ ) to the horizontal....[latexpage]

Spread the love

A projectile of mass 20.0 kg is fired at an angle of 55.0° to the horizontal with an initial velocity of 350 m/s. At the highest point of the trajectory, the projectile explodes into two equal fragments, one of which falls vertically downwards with no initial velocity immediately after the explosion. Neglect the effect of air resistance.

Explanation and Solution:

(i) How long after firing does the explosion occur?

The time to reach the highest point of the trajectory is given by:

$T = \frac{u \sin \alpha}{g}$

where $u = 350$ m/s (initial velocity), $\alpha = 55.0^\circ$ (angle of projection), and $g = 9.8$ m/s² (acceleration due to gravity).

Substituting the values:

$T = \frac{350 \sin 55^\circ}{9.8}$

$T = \frac{350 \times 0.8192}{9.8}$

$T = \frac{286.72}{9.8}$

$T \approx 29.25 \text{s}$

The explosion occurs 29.25 seconds after firing.

Final Answer: 29.25 seconds

(ii) Relative to the firing point, where do the two fragments hit the ground?

At the highest point of the trajectory, the velocity of the particle is entirely horizontal, being equal to $u \cos \alpha$ . The momentum of this particle at the highest point is $p = mu \cos \alpha$ , when $m$ is its mass. After the explosion, one fragment starts falling vertically and so does not carry any momentum initially. It would fall at half of the range, that is:

$R/2 = \frac{1}{2} \times \frac{u^2 \sin 2\alpha}{g}$

$= \frac{1}{2} \times \frac{(350)^2 \sin (2 \times 55^\circ)}{9.8}$

$\approx 5873 \text{ m}$

The second part of mass $\frac{1}{2}m$ proceeds horizontally from the highest point with initial momentum $p$ in order to conserve momentum. If its velocity is $v$ then:

$p = \frac{m}{2} v = mu \cos \alpha$

$v = 2u \cos \alpha = 2 \times 350 \cos 55^\circ$

$v = 401.5 \text{ m/s}$

Then its range will be:

$R' = v \sqrt{\frac{2h}{g}}$

But the maximum height:

$h = \frac{u^2 \sin^2 \alpha}{2g}$

Using this height in the range formula:

$R' = v \sqrt{\frac{2h}{g}}$

$R' = v \sqrt{\frac{2\times \frac{u^2 \sin^2 \alpha}{2g}}{g}}$

$R' = \frac{uv \sin\alpha}{g}$

$R'=\frac{350\times401.5 \times \sin 55^\circ}{9.8} \text{m}$

$R' \approx 11746 \text{ m}$

The distance from the firing point at which the second fragment hits the ground is:

$R/2 + R' = 5873 + 11746 = 17619 \text{ m}$

Final Answers:

First fragment: 5873 m from the firing point
Second fragment: 17619 m from the firing point

(iii) How much energy is released in the explosion?

Energy released is the difference between the kinetic energy of the fragments and the kinetic energy of the projectile at the time of the explosion:

$\text{Energy released} = \frac{1}{2} \times \frac{m}{2} v^2 - \frac{1}{2} m (u \cos \alpha)^2$

Substituting the values:

$\text{Energy released} = \frac{1}{2} \times 10 \times (401.5)^2 - \frac{1}{2} \times 20 \times (350 \times \cos 55^\circ)^2$

$\approx 4.03 \times 10^5 \text{ J}$

Final Answer: $4.03 \times 10^5$ J

Property	Value
Projectile mass	20.0 kg
Firing angle	55.0°
Time to explode	29.25 s
Explosion height	Not specified in the article
Fragment 1 velocity (vertical)	0 m/s
Fragment 1 velocity (horizontal)	0 m/s
Fragment 1 landing distance	5873 meters
Fragment 2 velocity (vertical)	0 m/s
Fragment 2 velocity (horizontal)	401.5 m/s
Fragment 2 landing distance	17619 meters
Explosion energy	$4.03\times 10^5$ J

A projectile of mass 20.0 kg is fired at an angle of $\Large 55.0^\circ$ to the horizontal….

Editorial Team

Leave a Reply Cancel reply

Editorial Team

You might also like

If the nth division of main scale coincides with (n+1)th division of vernier scale, find the least count of the vernier. Given one main scale divisions equal to ‘a’ units

A spring is used to stop a crate of mass 50 kg which is sliding on a horizontal surface. The spring has a spring constant k = 20kN/m and is initially in its equilibrium state….

A vernier calipers has 1mm marks on the main scale. It has 20 equal divisions on the Vernier scale which match with 16 main scale divisions. Calculate the least count of Vernier calipers.

Leave a Reply Cancel reply