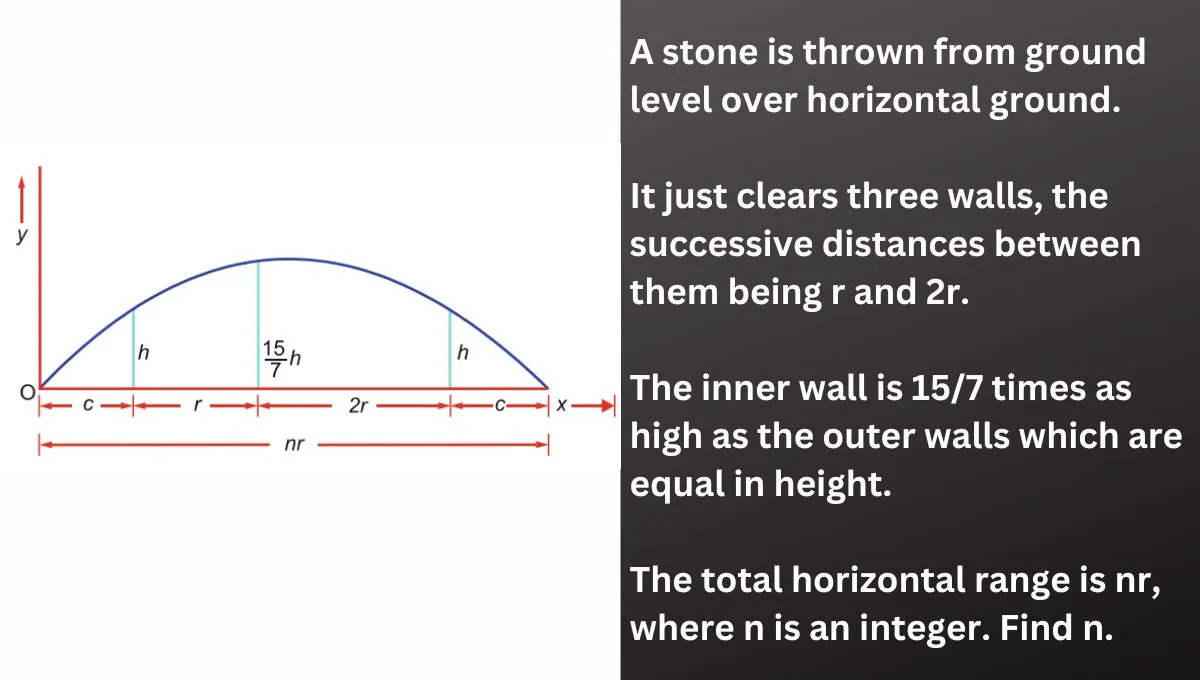
Cars A and B are travelling in adjacent lanes along a straight road...

Spread the love

Cars A and B are travelling in adjacent lanes along a straight road as shown.
At time, t = 0 their positions and speeds are as shown in the diagram. If car A
has a constant acceleration of $0.6 m/s^2$ and car B has a constant deceleration of
$0.46 m/s^2$ , determine when A will overtake B.

Property	Description
Cars	Car A and Car B
Location	Adjacent lanes on a straight road
Initial Conditions	* Positions and speeds of both cars at time t = 0 are specified in the article. * It’s assumed both cars travel in the same direction.
Motion	* Car A might have constant acceleration (positive or negative). * Car B might have constant acceleration (positive or negative) or constant velocity.
Unknown	The time (t) it takes for Car A to overtake Car B.
Solution Method	The article likely uses concepts of kinematics, including: * Equations for position and velocity considering constant acceleration. * Setting up an equation to determine when the relative position of Car A becomes greater than Car B (overtaking).

The problem involves two cars, A and B, traveling along a straight road with different accelerations. Car A starts at the origin with an unknown initial velocity and has a constant acceleration. Car B starts 30 meters ahead with a different initial velocity and a constant deceleration. The task is to determine the time at which Car A overtakes Car B.

Contents hide

1 Explanation:

2 Solution Steps:

3 Final Answer:

Explanation:

To solve this problem, kinematic equations for motion with constant acceleration are used. These equations relate the displacement (s), initial velocity (u), time (t), and acceleration (a) for an object in motion. By setting up an equation for each car’s motion and knowing that Car A overtakes Car B when they have traveled the same distance, the time at which this occurs can be determined.

Solution Steps:

Equation for Car A’s Motion:

Car A’s motion can be described by the equation

$s = ut + \dfrac{1}{2}at^2$

where $u$ is Car A’s initial velocity, $a$ is its acceleration, and $t$ is the time.

Car A’s acceleration $a$ is given as $0.6 m/s^2$ .

$s = 13t + \dfrac{1}{2}\times 0.6t^2$ –(1)

Equation for Car B’s Motion:

Car B’s motion, with initial position 30 m ahead of Car A and decelerating, is given by

$s - 30 = ut - \dfrac{1}{2}at^2$

where $s$ is the distance Car A travels, and $(s - 30)$ is the distance traveled by Car B.

Car B’s deceleration $a$ is given as $0.46 m/s^2$ (since deceleration is negative acceleration, it would be appropriate to use $-0.46 m/s^2$ in calculations).