The constant forces (Large{bf F_1 = hat i+2hat j +3hat k}) N and (Large{bf F_2 = 4hat i -5hat j -2hat k}) N act together on a particle...[latexpage]

Spread the love

Contents hide

1 Problem:

2 Solution:

2.1 Step 1: Calculate the total force

2.2 Calculation:

2.3 Step 2: Calculate the Displacement Vector

2.4 Calculation:

3 Final Answer:

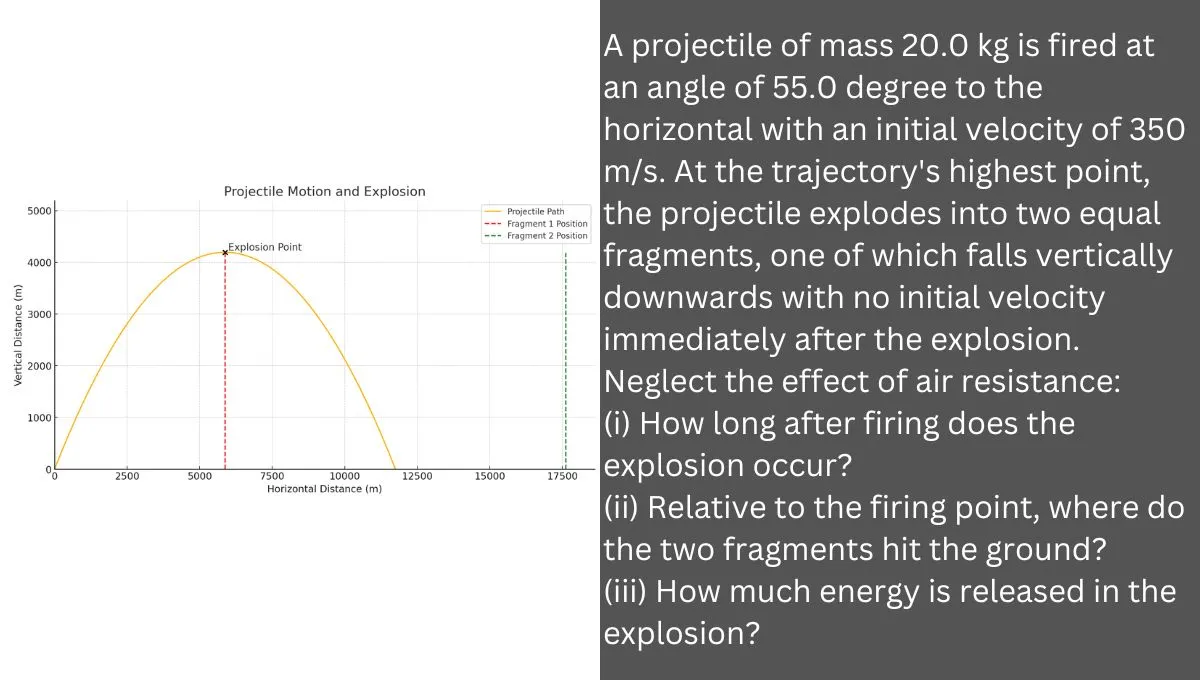
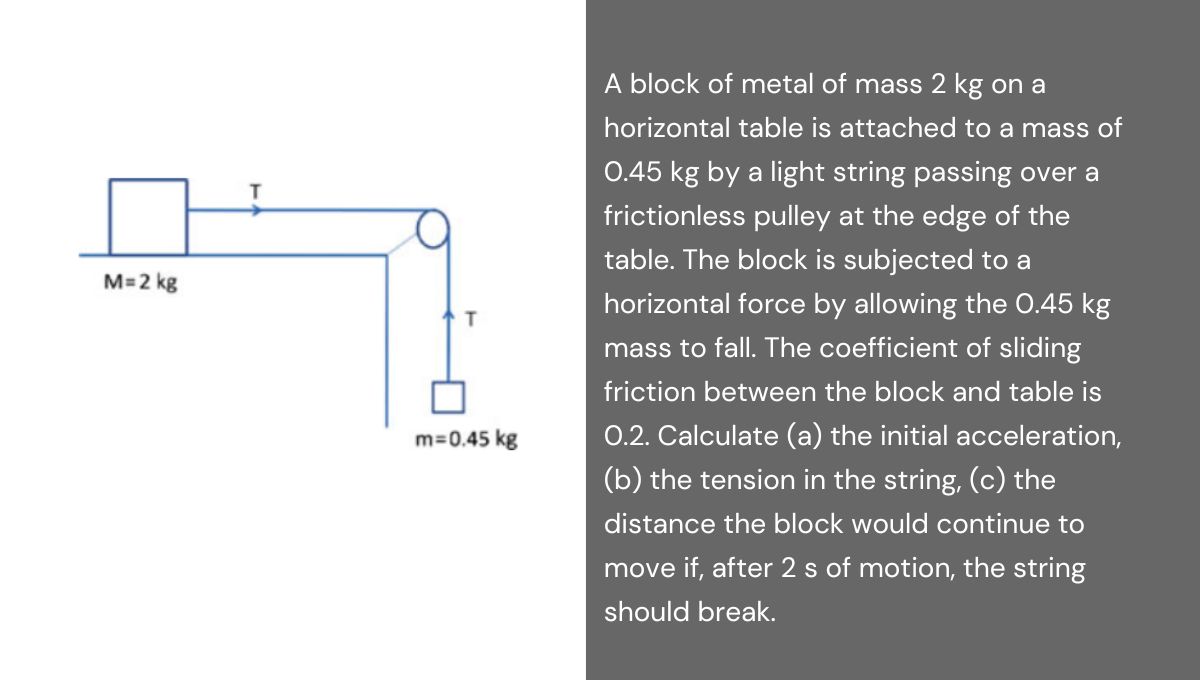
Problem:

The constant forces $\mathbf{F_1} = \hat{i} + 2\hat{j} + 3\hat{k} \, \text{N}$ and $\mathbf{F_2} = 4\hat{i} - 5\hat{j} - 2\hat{k} \, \text{N}$ act together
on a particle during a displacement from position $\mathbf{r_2} = 7\hat{k} \, \text{cm}$ to position $\mathbf{r_1} = 20\hat{i} + 15\hat{j} \, \text{cm}$ . Determine the total work done on the particle.

Solution:

Step 1: Calculate the total force

The total force $\mathbf{F}$ is the sum of the two forces:

$\mathbf{F} = \mathbf{F_1} + \mathbf{F_2}$

Calculation:

$\mathbf{F} &= (\hat{i} + 2\hat{j} + 3\hat{k}) + (4\hat{i} - 5\hat{j} - 2\hat{k})$

$= (\hat{i} + 4\hat{i}) + (2\hat{j} - 5\hat{j}) + (3\hat{k} - 2\hat{k})$

$= 5\hat{i} - 3\hat{j} + \hat{k} \, \text{N}$

Explanation: The components in the same directions ( $\hat{i}, \hat{j}, \hat{k}$ ) are added to get the total force.

Step 2: Calculate the Displacement Vector

The displacement vector $\mathbf{s}$ is obtained by subtracting the initial position from the final position:

$\mathbf{s} = \mathbf{r_1} - \mathbf{r_2}$

initial position:

$\mathbf{r_2} = 0\hat{i} + 0\hat{j} + 7\hat{k} \, \text{cm}$

Final position:

$\mathbf{r_1} = 20\hat{i} + 15\hat{j} + 0\hat{k} \, \text{cm}$

Therefore,

$\mathbf{s} &= (20\hat{i} + 15\hat{j} + 0\hat{k}) - (0\hat{i} + 0\hat{j} + 7\hat{k})$

$= (20 - 0)\hat{i} + (15 - 0)\hat{j} + (0 - 7)\hat{k}$

$= 20\hat{i} + 15\hat{j} - 7\hat{k} \, \text{cm}$

Convert this to meters (since the force is in Newtons):

$\mathbf{s} = 0.20\hat{i} + 0.15\hat{j} - 0.07\hat{k} \, \text{m}$

Explanation: The displacement vector shows the direction and distance in which the particle is displaced.

You may also want to know about projectile motion.

Step 3: Calculate the work

The formula for work $W$ is:

$W = \mathbf{F} \cdot \mathbf{s}$

Here “ $\cdot$ ” represents the dot product.

Calculation:

$W &= (5\hat{i} - 3\hat{j} + \hat{k}) \cdot (0.20\hat{i} + 0.15\hat{j} - 0.07\hat{k})$

$= (5 \times 0.20) + (-3 \times 0.15) + (1 \times -0.07)$

$= 1.00 - 0.45 - 0.07 \\&= 0.48 \, \text{Joule}$

Explanation: In dot product, components in same directions are multiplied and then added. This ensures correct calculation of work as work is scalar product between force and displacement.

Final Answer:

The total work is $\bf 0.48$ joules.

References:

The constant forces $\Large{\bf F_1 = \hat i+2\hat j +3\hat k}$ N and $\Large{\bf F_2 = 4\hat i -5\hat j -2\hat k}$ N act together on a particle…

Problem:

Solution:

Step 1: Calculate the total force

Calculation:

Step 2: Calculate the Displacement Vector

Calculation:

Final Answer:

Leave a Comment Cancel Reply

Problem:

Solution:

Step 1: Calculate the total force

Calculation:

Step 2: Calculate the Displacement Vector

Calculation:

Final Answer:

Related Posts

Leave a Comment Cancel Reply