by Table: Main Components of Torque
| Components | Description |
|---|---|
| Torque (τ) | Rotational effect produced in an object due to a force |
| Force (F) | External force applied on the object |
| Distance (r) | Perpendicular distance from the point of force to the axis of rotation |
| Angle (θ) | Angle between force and distance vector |
| Formula | τ = r × F × sinθ |
| Unit | Newton-meter (N m) |
In physics, torque is an important concept that deals with rotational motion and equilibrium. It is essential to understand how force and the distance from the point of its application to the axis of rotation affect the rotation of an object.
What is torque?
Torque, also known as rotational force, is the measurement that shows the ability of an object to rotate or turn. When we apply force to an object, and that force is applied at a distance from the object’s axis of rotation, that object can start rotating. For example, when we press a door handle, the door opens or closes.
Mathematical Representation of Torque
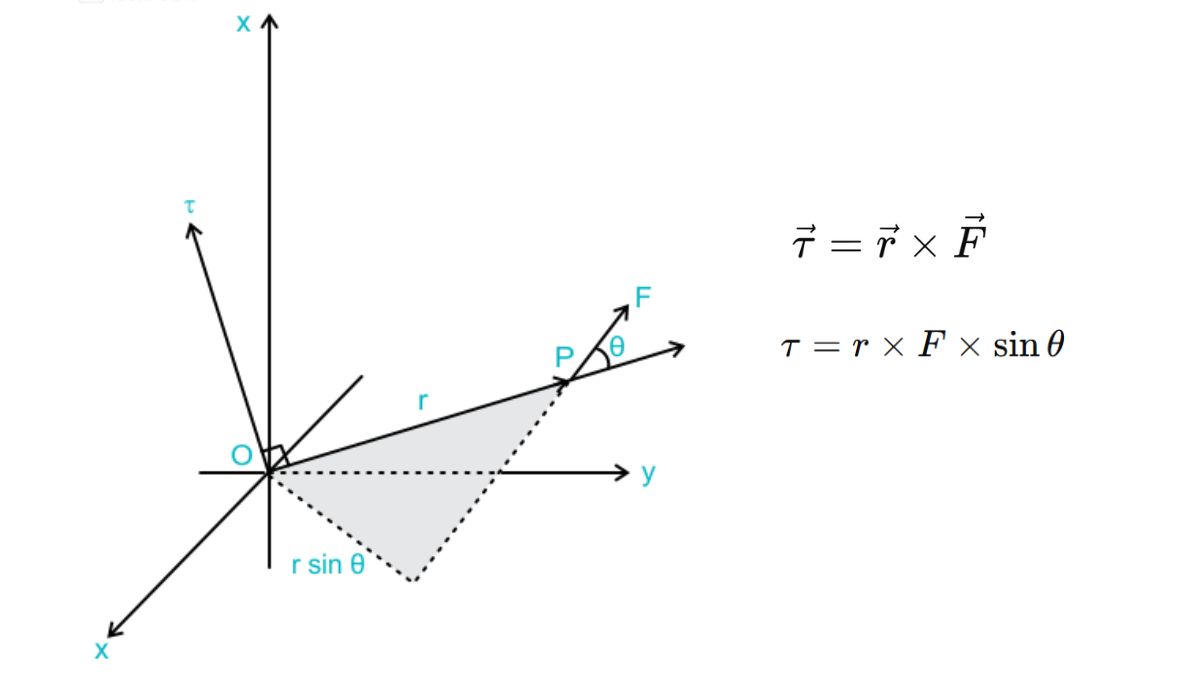
The mathematical formula for torque is:
![\[ \tau = r \times F \times \sin\theta \]](https://physicsqanda.com/wp-content/ql-cache/quicklatex.com-bc5e07aa099690c7b14b1c3dcdff3e7e_l3.png "Rendered by QuickLaTeX.com")
where:
τ (torque) is the rotational effect produced in the object,
r is the perpendicular distance from the point of force to the axis of rotation,
F is the force applied,
θ is the angle between the force and distance vector.
This formula shows that torque depends on force, distance, and the angle between them.
Right-hand rule
The right-hand rule is used to determine the direction of torque. Move the fingers of your right hand from r to F; your thumb will show the direction of torque.
Practical examples of torque
- Using a screwdriver
When we tighten a screw with a screwdriver, we apply torque. The force applied to the handle and the radius of the handle produce torque that turns the screw.
- Opening or closing a door
Applying force to a door handle produces torque, which causes the door to rotate around its hinges. The farther the handle is, the less force is required.
- Pedaling a bicycle
Applying force to the pedals of a bicycle produces torque on the chain and wheels, which causes the bicycle to move forward. The length of the pedals and cranks affect torque.
Torque and equilibrium
An object is in equilibrium when the sum of all torques applied to it is zero. It is essential for mechanical equilibrium. For example, a bridge needs to have a balance of all forces and torques applied to it so that it can safely carry the load of vehicles.
Static equilibrium
When an object is neither moving nor rotating, it is in static equilibrium. This requires that:
The total force is zero:
![\[ \sum F = 0 \]](https://physicsqanda.com/wp-content/ql-cache/quicklatex.com-fab28d93eaece91d395158ac1c1c9bec_l3.png "Rendered by QuickLaTeX.com")
The total torque is zero:
![\[ \sum \tau = 0 \]](https://physicsqanda.com/wp-content/ql-cache/quicklatex.com-4e14dd4353155507459a1f1bb0c37693_l3.png "Rendered by QuickLaTeX.com")
Importance of torque
In engineering
Understanding torque is important in the design of machines, vehicles, and structures. The torque of an engine determines its capacity and performance. Engines with high torque are able to lift heavier loads.
In sports
Players use torque while throwing a ball in cricket or kicking a ball in football to give the ball the required speed and direction. In gymnastics, the players’ bodies balance torque and moment of inertia while rotating.
In daily life
Many household appliances such as blenders, mixers, and drills depend on torque. The torque of their motors determines their efficiency. Torque is also involved in tasks such as opening a door, closing a tap, etc.
Angular Momentum
Torque is the rate of change of angular momentum. It is the rotational version of Newton’s second law.
![\[ \tau = \dfrac{dL}{dt} \]](https://physicsqanda.com/wp-content/ql-cache/quicklatex.com-a8056c5e6c0998e42c0a03ccdf40803e_l3.png "Rendered by QuickLaTeX.com")
Where L is the angular momentum. This principle states that if no external torque is applied to an object, its angular momentum is conserved.
Moment of Inertia
It is a measure of the mass distribution of an object. If the moment of inertia of the object is high, more torque is required to bring it into rotation.
![\[ \tau = I \times \alpha \]](https://physicsqanda.com/wp-content/ql-cache/quicklatex.com-8fd0b0876c9bef34f2e918f97d0fb257_l3.png "Rendered by QuickLaTeX.com")
Where:
I is the moment of inertia,
α is the angular acceleration.
This formula shows that torque is equal to the product of moment of inertia and angular acceleration.
Uses of Torque
In Machinery
Management of torque is important in gear systems. Torque and speed are controlled by changing gear ratios. High torque is required in heavy machinery.
In Architecture
In the design of buildings and bridges it is necessary to ensure balance of torques and forces so that the structure remains stable. Special designs are made to protect buildings from torques produced due to wind and earthquakes.
Mathematical Problems
Example Questions
Question: A force of 50 Newton is applied vertically at the end of a 2 meter long wrench. Find the torque produced.
Answer:
Since the force is perpendicular, θ = 90°, then sin90° = 1
![\[ \tau = 2 \times 50 \times 1 = 100 \text{ N·m} \]](https://physicsqanda.com/wp-content/ql-cache/quicklatex.com-04b6966bfde295aabbdfe2c51da92f40_l3.png "Rendered by QuickLaTeX.com")
Conclusion
Torque is a fundamental concept in physics which deals with rotational motion, operation of machines,and helps in understanding many aspects of daily life. Its understanding is important in scientific and engineering fields.
Additional Resources
Books:
“Fundamentals of Physics” – by Halliday, Resnick and Walker
“Concepts of Physics” – by H.C. Verma
Websites:
Khan Academy – Online Learning Materials
Physics World – Latest Research and Articles
