(a) Figure shows two point clusters of charge situated in free space placed on a line that is called the x-axis. The first, with a positive charge of Q1 =+8e, is at the origin......

Spread the love

Contents hide

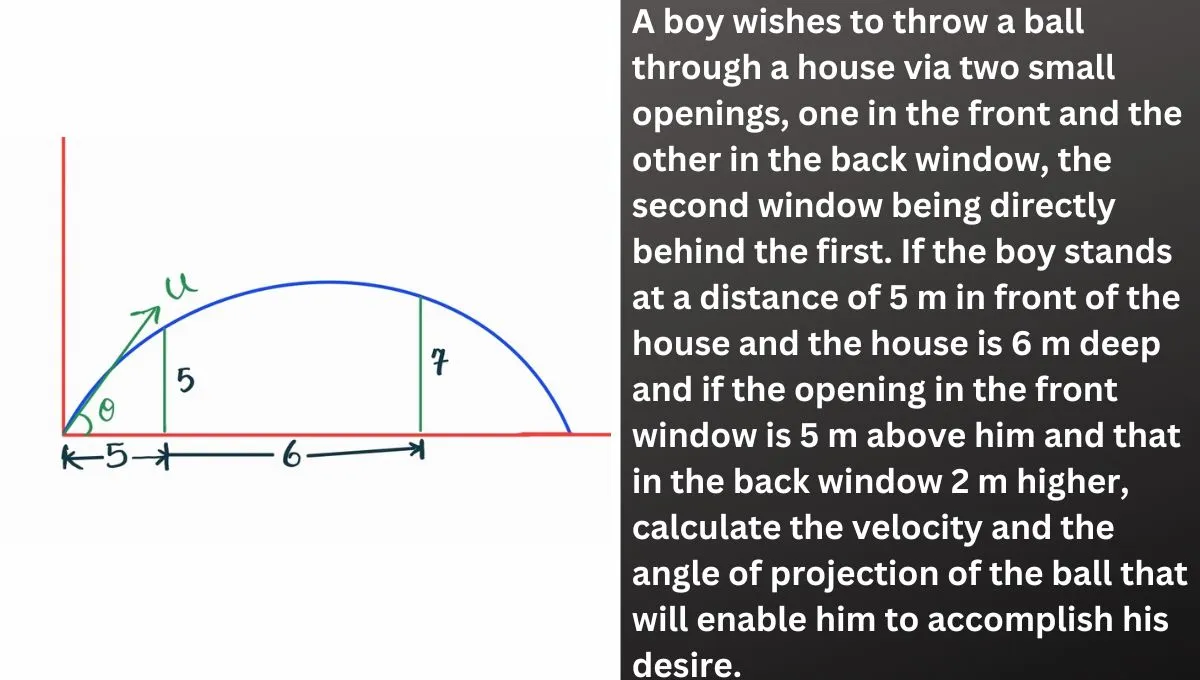
Problem:

(a) Figure shows two point clusters of charge situated in free space placed on a line that is called the x-axis. The first, with a positive charge of $Q_1 =+8e$ , is at the origin. The second, with a negative charge of $Q_2 =−4e$ ,is to the right at a distance equal to 0.2m.
(i) What is the magnitude of the force between them?
(ii) Where would you expect to find the position of zero electric field: to the left of $Q_1$ , between $Q_1$ and $Q_2$ or to the right of $Q_2$ ? Briefly explain your choice and then work out the exact position.
(b) The electron in a hydrogen atom orbits the proton at a radius of $5.3\times 10^{-11}$ m.
(i) What is the proton’s electric field strength at the position of the electron?
(ii) What is the magnitude of the electric force on the electron?

Explanation:

For part (a):
(i) The electric force between two charges is calculated using Coulomb’s law:

$F = k_e \dfrac{|Q_1 Q_2|}{r^2}$

where $k_e = 8.99 \times 10^9 \, \text{N·m}^2/\text{C}^2$ is the Coulomb constant, and $e = 1.6 \times 10^{-19} \, \text{C}$ is the elementary charge.

(ii) The electric field is zero at a point where the field contributions from both charges cancel. This occurs closer to the smaller charge because the electric field’s magnitude decreases with distance.

For part (b):
(i) The electric field strength due to a charge is given by:

$E = k_e \dfrac{|Q|}{r^2}$

where $Q = e = 1.6 \times 10^{-19} \, \text{C}$ .

(ii) The electric force acting on a charge in an electric field is given by:

$F = qE$

where $q = -e = -1.6 \times 10^{-19} \, \text{C}$ .

Solution:

Part (a):

(i) Force between $Q_1$ and $Q_2$ :

$Q_1 = +8e = 8 \times 1.6 \times 10^{-19}$

$= 1.28 \times 10^{-18} \, \text{C}$

$Q_2 = -4e = -4 \times 1.6 \times 10^{-19}$

$= -6.4 \times 10^{-19} \, \text{C}$

$F = k_e \dfrac{|Q_1 Q_2|}{r^2}$

$= (8.99 \times 10^9) \dfrac{(1.28 \times 10^{-18})(6.4 \times 10^{-19})}{(0.2)^2}$

$F = (8.99 \times 10^9) \dfrac{8.192 \times 10^{-37}}{0.04}$

$F = (8.99 \times 10^9)(2.048 \times 10^{-35}) \[= 1.841 \times 10^{-25} \, \text{N}$

Final Answer: $F = 1.841 \times 10^{-25} \, \text{N}$

(ii) Position of zero electric field:
The zero-field point lies between $Q_1$ and $Q_2$ because the charges have opposite signs. Let the distance of the zero-field point from $Q_1$ be $x$ . Then the distance from $Q_2$ is $(0.2 - x)$ .

The electric field at the zero point due to $Q_1$ and $Q_2$ must be equal in magnitude:

$E_1 = E_2 \quad \Rightarrow \quad \dfrac{k_e |Q_1|}{x^2} = \dfrac{k_e |Q_2|}{(0.2 - x)^2}$

$\dfrac{|Q_1|}{x^2} = \dfrac{|Q_2|}{(0.2 - x)^2} \quad \Rightarrow \quad \dfrac{8}{x^2} = \dfrac{4}{(0.2 - x)^2}$

$2 = \dfrac{(0.2 - x)^2}{x^2} \quad \Rightarrow \quad 2x^2 = (0.2 - x)^2$

$2x^2 = 0.04 - 0.4x + x^2 \quad \Rightarrow \quad x^2 + 0.4x - 0.04 = 0$

Solving this quadratic equation:

$x = \dfrac{-b \pm \sqrt{b^2 - 4ac}}{2a} = \dfrac{-0.4 \pm \sqrt{(0.4)^2 - 4(1)(-0.04)}}{2(1)}$

$x = \dfrac{-0.4 \pm \sqrt{0.16 + 0.16}}{2} = \dfrac{-0.4 \pm 0.5657}{2}$

$x = \dfrac{0.1657}{2} = 0.08285 \, \text{m} \quad \text{(discarding negative root)}$

Final Answer: The zero electric field is at $x = 0.08285 \, \text{m}$ from $Q_1$ .

Part (b):

(i) Electric field strength at the electron’s position:

$E = k_e \dfrac{|Q|}{r^2} = (8.99 \times 10^9) \dfrac{1.6 \times 10^{-19}}{(5.3 \times 10^{-11})^2}$

$E = 5.12 \times 10^{11} \, \text{N/C}$

Final Answer: $E = 5.12 \times 10^{11} \, \text{N/C}$

(ii) Electric force on the electron:

$F = qE = (1.6 \times 10^{-19})(5.12 \times 10^{11}) = 8.19 \times 10^{-8} \, \text{N}$

Final Answer: $F = 8.19 \times 10^{-8} \, \text{N}$

(a) Figure shows two point clusters of charge situated in free space placed on a line that is called the x-axis. The first, with a positive charge of Q1 =+8e, is at the origin……

Problem:

Explanation:

Solution:

Part (a):

Part (b):

admin

Leave a Reply Cancel reply

Problem:

Explanation:

Solution:

Part (a):

Part (b):

admin

You might also like

A vernier calipers has 1mm marks on the main scale. It has 20 equal divisions on the Vernier scale which match with 16 main scale divisions. Calculate the least count of Vernier calipers.

If the nth division of main scale coincides with (n+1)th division of vernier scale, find the least count of the vernier. Given one main scale divisions equal to ‘a’ units

A stone is thrown from ground level over horizontal ground. It just clears three walls..

Leave a Reply Cancel reply