A spring is used to stop a crate of mass 50 kg which is sliding on a horizontal surface. The spring has a spring constant k = 20kN/m and is initially in its equilibrium state….

By /
Spread the love
Contents hide
1 Problem:
2 Explanation:
3 Solution:
3.1 (i) Work Done by the Spring:
3.2 (ii) Work Done by Friction:
3.3 (iii) Coefficient of Friction:
3.4 (iv) Velocity of the Crate as it Passes Position A After Rebounding:

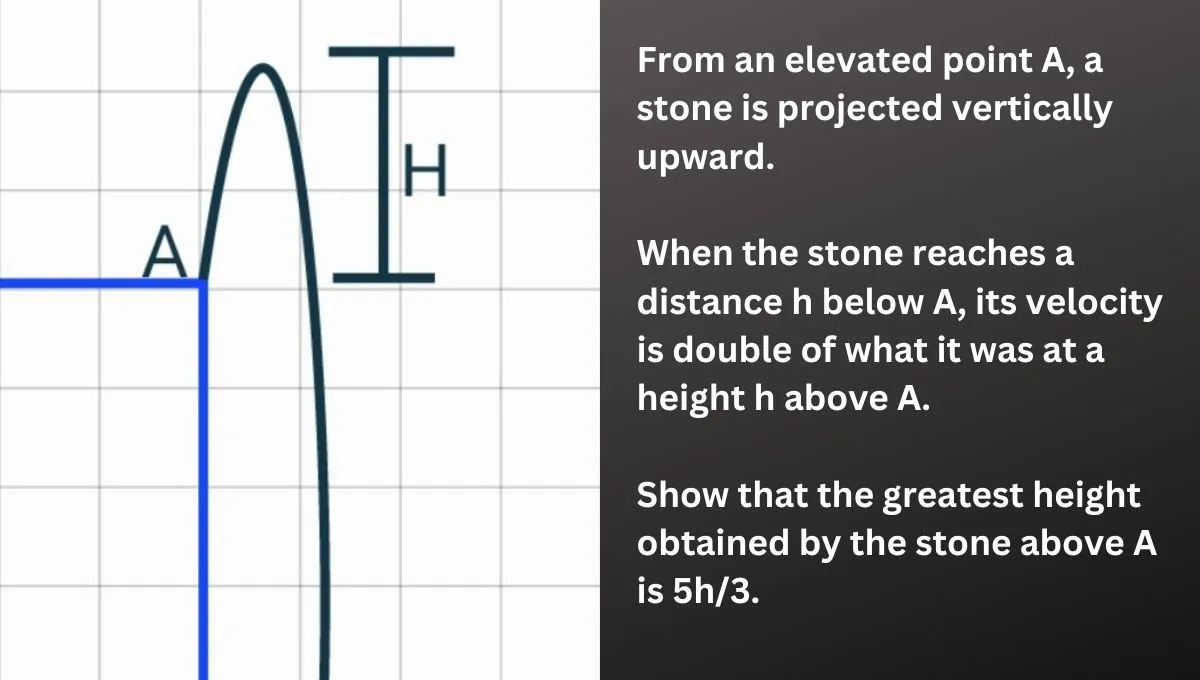
Problem:

A spring is used to stop a crate of mass 50 kg which is sliding on a horizontal
surface. The spring has a spring constant k = 20kN/m and is initially in its
equilibrium state. In position A shown in the top diagram the crate has a veloc-
ity of 3.0 m/s. The compression of the spring when the crate is instantaneously
at rest (position B in the bottom diagram) is 120 mm.

(i) What is the work done by the spring as the crate is brought to a stop?
(ii) Write an expression for the work done by friction during the stopping of
the crate (in terms of the coefficient of kinetic friction).
(iii) Determine the coefficient of friction between the crate and the surface.
(iv) What will be the velocity of the crate as it passes again through position
A after rebounding off the spring(image (a) (b))?

Explanation:

  1. The spring does work on the crate as it compresses, storing elastic potential energy in the spring. This work can be calculated using the spring energy formula.
  2. Friction opposes the motion of the crate, doing negative work over the distance it travels.
  3. The coefficient of friction can be determined using the relationship between work done by friction, the weight of the crate, and the distance traveled.
  4. When the crate rebounds, it gains kinetic energy, which can be related to the energy lost due to friction and the energy stored in the spring.

You may also want to know about Fluids.

Solution:

(i) Work Done by the Spring:

The work done by the spring is given by:

    \[W_s = -\frac{1}{2}kx^2\]


Substituting the values:

    \[W_s = -\frac{1}{2} \times 20,000 \times (0.12)^2\]


    \[W_s = -144 \, \text{J}\]

(ii) Work Done by Friction:


The total work done on the crate is equal to the change in its kinetic energy:

    \[W_{\text{total}} = \Delta KE = \frac{1}{2} m v^2 - 0\]


Substituting:

    \[\Delta KE = \frac{1}{2} \times 50 \times (3.0)^2 = 225 \, \text{J}\]


The work done by friction is:

    \[W_f = W_{\text{total}} - W_s\]


    \[W_f = 225 - 144 = 81 \, \text{J}\]

(iii) Coefficient of Friction:


The work done by friction can also be expressed as:

    \[W_f = \mu m g s\]


Rearranging for ( \mu ):

    \[\mu = \frac{W_f}{m g s}\]


The distance traveled is s = 0.60 + 0.12 = 0.72 \, \text{m}. Substituting:

    \[\mu = \frac{81}{50 \times 9.8 \times 0.72}\]


    \[\mu = 0.2296\]

(iv) Velocity of the Crate as it Passes Position A After Rebounding:


The velocity of the crate after rebounding can be determined using:

    \[\frac{1}{2} m v_1^2 = W_s - \mu m g s\]


Rearranging for v_1:

    \[v_1^2 = \frac{2}{m} (W_s - \mu m g s)\]


Substituting the values:

    \[v_1^2 = \frac{2}{50} \times (144 - 0.2296 \times 50 \times 9.8 \times 0.72)\]


    \[v_1^2 = \frac{2}{50} \times 63\]


    \[v_1^2 = 2.52\]


    \[v_1 = \sqrt{2.52}\]


    \[v_1 = 1.587 \, \text{m/s}\]

Final Answers:

  1. Work done by the spring: W_s = 144 \, \text{J}
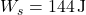
  2. Work done by friction: W_f = 81 \, \text{J}
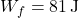
  3. Coefficient of friction: \mu = 0.2296
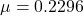
  4. Velocity of the crate after rebounding: v_1 = 1.587 \, \text{m/s}

Related Posts

Leave a Comment

Your email address will not be published. Required fields are marked *

Scroll to Top