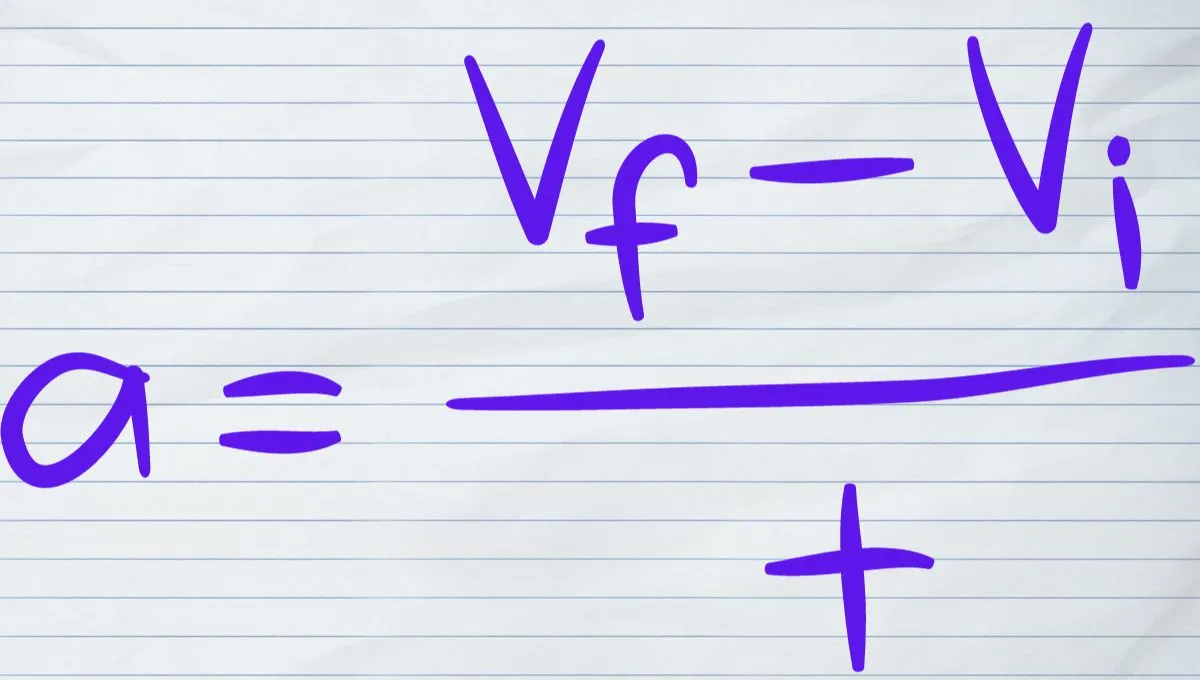How To Find Instantaneous Velocity

Spread the love

To find the instantaneous velocity of an object, we have to use the concept of limits and derivatives(calculus). Instantaneous velocity is defined as the velocity of an object at any instant of time or at a specific point in its path.

\lim_{\Delta t \to 0} \dfrac{\Delta x}{\Delta t} = \dfrac{dx(t)}{dt}

Contents hide

1 Derivation of formula of instantaneous velocity

2 Step-by-step Guide To Calculate Instantaneous velocity From Displacement Function

3 Step-by-step Guide To Calculate Instantaneous velocity From s-t curve

4 Examples:

5 FAQs:

5.1 What speedometer reads?

5.2 Magnitude of average velocity is average speed?

Derivation of formula of instantaneous velocity

Understanding Average Velocity: First calculate the average velocity over a time interval. Average velocity is the displacement (change in position) divided by the time taken for that displacement. It is given by the formula:

$\text{Average Velocity} = \frac{\Delta x}{\Delta t}$

where $\Delta x$ is the change in position and $\Delta t$ is the change in time.

Decreasing the Time Interval: To find the instantaneous velocity, gradually reduce the time interval ( $\Delta t$ ) over which the average velocity is calculated. This process involves considering smaller and smaller intervals of time around the instant for which the velocity is desired.

Applying the Limit Concept: Instantaneous velocity is the limit of the average velocity as the time interval approaches zero. In mathematical terms, it is expressed as:

$v = \lim_{{\Delta t \to 0}} \frac{\Delta x}{\Delta t}$

Using Derivatives: In calculus, the instantaneous velocity is the derivative of the position function with respect to time. If $x(t)$ is the position of the object as a function of time, the instantaneous velocity $v$ at time $t$ is:

$v = \frac{dx(t)}{dt}$

This derivative represents the rate of change of position with respect to time at any given instant.

Finally we arrive at the formula:

$\boxed{v_{inst} = \underset{\Delta t \to 0}{Lim} \dfrac{\Delta x(t)}{\Delta t} = \dfrac{d x(t)}{dt}}$

Step-by-step Guide To Calculate Instantaneous velocity From Displacement Function

If displacement is given as a function of time, the instantaneous velocity can be calculated using calculus. The instantaneous velocity is the derivative of the displacement function with respect to time. Here are the steps:

Understand the Displacement Function:
The displacement of an object is given as a function of time, often denoted as $s(t)$ , where $s$ is the displacement and $t$ is the time.
Differentiate the Displacement Function:
Find the first derivative of the displacement function with respect to time. This derivative, denoted as $s'(t)$ or $\frac{ds}{dt}$ , represents the velocity function $v(t)$ .
Evaluate the Derivative at the Specific Time:
To find the instantaneous velocity at a particular time $t = t_0$ , substitute $t_0$ into the velocity function. This will give you the value of the instantaneous velocity at that specific moment.
Interpret the Result:
The resulting value is the instantaneous velocity of the object at time $t_0$ . If you get a positive value, it means the object is moving in the positive direction of the axis, and a negative value indicates movement in the negative direction.

Here’s an example to illustrate the process:

Suppose the displacement of an object is given by the function $s(t) = 4t^2 - 3t + 2$ , and you want to find the instantaneous velocity at $t = 5$ seconds.

Step 1: The displacement function is $s(t) = 4t^2 - 3t + 2$ .

Step 2: Differentiate $s(t)$ with respect to $t$ to get the velocity function:

$v(t) = s'(t) = \frac{d}{dt}(4t^2 - 3t + 2) = 8t - 3$

Step 3: Evaluate $v(t)$ at $t = 5$ :

$v(5) = 8(5) - 3 = 40 - 3 = 37$

Step 4: The instantaneous velocity at $t = 5$ seconds is $37$ units of distance per unit of time in the positive direction.

Step-by-step Guide To Calculate Instantaneous velocity From s-t curve

To calculate the instantaneous velocity from a position-time (s-t) curve, follow these steps:

Plot or Obtain the s-t Curve:
Ensure you have a position-time graph where the object’s position $s$ is plotted against time $t$ . The curve should be smooth and continuous to accurately determine the instantaneous velocity.
Identify the Point of Interest:
Decide the specific time $t_0$ at which you want to find the instantaneous velocity. This is the point on the curve where you will calculate the slope.
Draw a Tangent Line:
At the point of interest on the $s-t$ curve, draw a tangent line. This line should just touch the curve at that point without cutting through it elsewhere.
Determine Two Points on the Tangent Line:
Select two points on the tangent line, ideally as far apart as possible to minimize the error in your slope calculation. The points can be labeled as $(t_1, s_1)$ and $(t_2, s_2)$ .
Calculate the Slope of the Tangent Line:
Use the standard slope formula for a line, which is the change in position divided by the change in time:
$\text{slope} = \frac{\Delta s}{\Delta t} = \frac{s_2 - s_1}{t_2 - t_1}$

This slope represents the instantaneous velocity at $t_0$ .
Analyze the Slope:
The slope you’ve calculated from the tangent line gives you the instantaneous velocity at time $t_0$ . If the slope is positive, the velocity is positive, indicating that the position is increasing with time. If the slope is negative, the velocity is negative, meaning the position is decreasing with time.
Units and Direction:
Ensure that the units of velocity are consistent with the units used for position and time on the $s-t$ graph. Also, take note of the direction: a velocity-time graph might indicate direction with positive and negative values, so interpret the sign of your result accordingly.

These steps will yield the instantaneous velocity at a specific time from a position-time curve. This process assumes that you have a clear and precise curve from which to work. In cases where the curve is not smooth, or it’s difficult to draw a precise tangent, you may need to use more advanced mathematical techniques or software tools that can calculate the derivative at a point more accurately.

Here’s an example to illustrate the process:

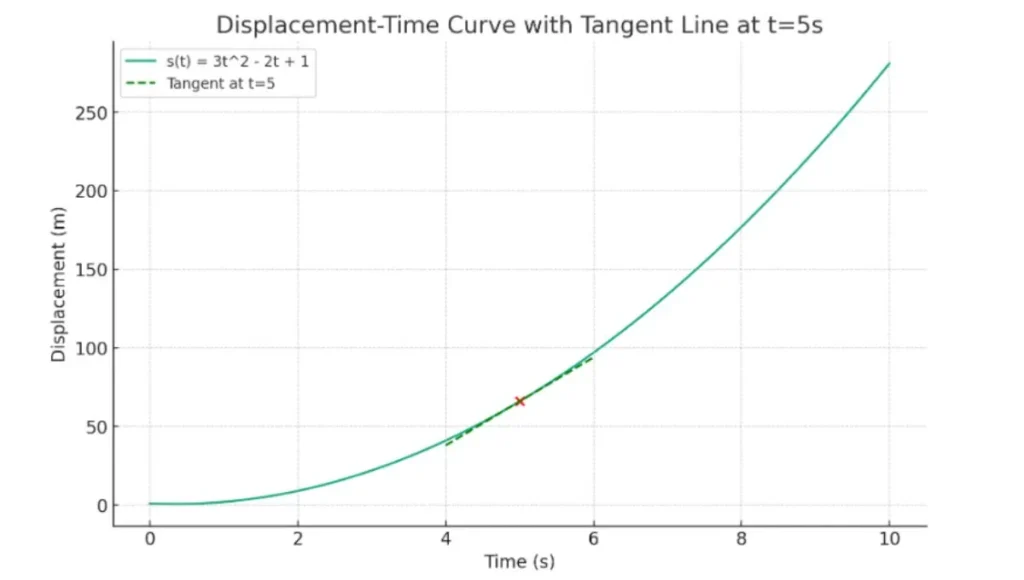
The plot above represents the displacement-time (s-t) curve for the function $s(t) = 3t^2 - 2t + 1$ .

$\text{slope} = \frac{\Delta s}{\Delta t} = \frac{s_2 - s_1}{t_2 - t_1}$

$\text{slope} = \frac{(3(6)^2 - 2(6) + 1) - (3(4)^2 - 2(4) + 1)}{6 - 4} = 28$

This slope is at $t=5s$ represents the instantaneous velocity.

The result is an instantaneous velocity of $28$ units of distance per unit of time (e.g., 28 m/s if the units are meters and seconds).

Examples:

(1) The displacement of an object as a function of time if given by the function, $x(t) = (12t^2+4t+9)m$ .Calculate the instantaneous velocity at $t = 3 s$ .

The displacement function given is:

$x(t) = 12t^2 + 4t + 9$

The velocity function $v(t)$ is the first derivative of the displacement function with respect to time:

$v(t) = \frac{dx}{dt}$

$v(t) = \frac{d(12t^2 + 4t + 9)}{dt}$

$v(t) = \frac{d(12t^2)}{dt} +\frac{d(4t)}{dt} + \frac{d(9)}{dt}$

Now, we’ll calculate the first derivative step-by-step:

Take the derivative of the first term $12t^2$ . The derivative of $t^2$ with respect to $t$ is $2t$ , so the derivative of $12t^2$ is $12 \times 2t = 24t$ .
Take the derivative of the second term $4t$ . The derivative of $t$ with respect to $t$ is 1, so the derivative of $4t$ is $4 \times 1 = 4$ .
Take the derivative of the constant term $9$ . The derivative of a constant is zero.

Putting it all together, we have the velocity function:

$v(t) = 24t + 4 + 0$

To find the instantaneous velocity at $t = 3$ seconds, we substitute $t$ with 3 in the velocity function:

$v(3) = 24(3) + 4$

$v(3) = 72 + 4$

$v(3) = 76$

Therefore, the instantaneous velocity at $t = 3$ seconds is $\bf{76}$ meters per second.

(2) A particle moving along straight line according to the equation $t =\sqrt{1+x}$ . Calculate instantaneous velocity at $t = 2s$ .

The equation for the of the path is $t = \sqrt{1 + x}$

Squaring both the side,

$t^2 = 1 + x$

Rearrange this equation,

$x = t^2 - 1$

Differentiate this to get the function of velocity

$\dfrac{dx}{dt} = \dfrac{d(t^2)}{dt} -\dfrac{d(1)}{dt}$

$v_{inst} = 2t - 0$

at $t=2s$

$v_{|t=2} =2(2) = 4 m/s$

FAQs:

What speedometer reads?

Speedometer reads instantaneous speed.

Magnitude of average velocity is average speed?

In general, average speed is not equal to the magnitude of average velocity, but it can be so, in case of straight line motion without change in direction.

Topic	Description
Definition	Instantaneous velocity is the velocity of an object at a specific moment in time. It is the derivative of the position function with respect to time.
Formula Derivation	Average velocity: $\dfrac{\Delta x}{\Delta t}$ Instantaneous velocity: $\lim_{\Delta t \to 0} \dfrac{\Delta x}{\Delta t} = \dfrac{dx(t)}{dt}$
Displacement Function	To find instantaneous velocity from a displacement function (s(t)): 1. Differentiate $s(t)$ to get $v(t)$ . 2. Evaluate $v(t)$ at the specific time $t_0$ .
s-t Curve Method	Steps to find instantaneous velocity from an s-t curve: 1. Draw the tangent line at the desired point. 2. Calculate the slope of the tangent.
Examples	1. For $s(t) = 4t^2 - 3t + 2$ at $t = 5$ , $v(5) = 37$ . 2. For $t = \sqrt{1+x}$ at $t = 2$ , $v = 4 \text{ m/s}$ .